Retrieval of lost reflections in high resolution Fourier syntheses by a ‘soft’ solvent flattening.

 

by Natalia L. Lunina¹, Vladimir Y. Lunin¹ & Alberto D. Podjarny²

 

¹Institute of Mathematical Problems of Biology, Russian Academy of Sciences, Pushchino, Moscow region, 142290 Russia; lunina@impb.psn.ru .

²UPR de Biologie Structurale, IGBMC, BP 163, 67404 Illkirch, CU Strasbourg, France, podjarny@igbmc.u-strasbg.fr.

 

1. Abstract.

 

            Accurate studies of a high resolution Fourier synthesis require the full set of structure factors to be used when calculating the synthesis. Structure factors with unknown phase or even with unknown amplitude may be restored with a reasonable accuracy through density modification methods. For the case of Aldose Reductase, measured at 0.9 A resolution, a special type of the solvent flattening was tested for restoring about 20 000 (10% of the full set) structure factors in 0.9Å resolution zone. This flattening is based on the connectivity analysis of the Fourier synthesis map and is applied to small ‘drops’ only.

 

2. Model-free structure factors retrieval.

 

The quality of Fourier syntheses maps depend both on the accuracy of magnitude and phase values of structure factors and on the completeness of the set of structure factors used to calculate the map. The impact of a relatively small number of lost reflections on the quality of low resolution syntheses was demonstrated in a number of papers and different ways to restore these lost values were discussed (Podjarny, Schevitz & Sigler, 1981; Lunin, 1988; Lunin & Skovoroda, 1991; Urzhumtsev, 1991). Figs. 1-3 give some examples of such restoring.

 

 

 

Fig.1. Comparison of ten sections of the 4.5Å map of yeast tRNA before (a) and after (b) the inclusion of 28 low-resolution terms whose phases were determined by matrical methods (Podjarny, Schevitz & Sigler (1981), Acta Cryst. A37, 662-668).

 

 

 

 

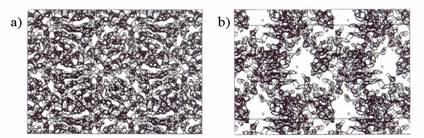
Fig. 2. (a) Initial (SIR) 8Å map for the elongation factor G and (b) the map with additional 29 low resolution reflections restored by the Double Step Filtration method (Urzhumtsev (1991), Acta Cryst. A47, 794-801.

 

 

 

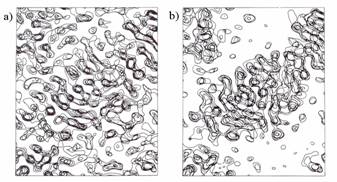
Fig. 3. (a) Initial 4 Å map for g-crystallin IIIb and (b) the map with additional structure factors restored from electron-density histogram (Lunin & Skovoroda (1991), Acta Cryst., A47, 45-52.

 

The importance of complete data sets is not restricted to low resolution only, but is also true at subatomic resolution, where the electron density distribution in itself becomes the subject for studies. The missing reflections can be important when investigating these fine features at low contour levels. At the same time, the positions of peaks in high resolution Fourier syntheses are less sensible to missing reflections and may be found correctly even when some structure factors are absent (see Fig.5, 6 for comparison). 

The retrieval of the lost structure factors may consist in either determination of their phases (if the reflection magnitude was measured, but was not phased) or restoring both the magnitude and the phase of structure factor (if the reflection was not measured). We discuss below the possibility of restoring both magnitudes and phases of the lost structure factors in the ‘nominal’ resolution zone. The extrapolation of data to higher resolution zones (Karle & Hauptman, 1964; Langs, 1998; Xu & Hauptman, 2000) is out of the scope of these notes. In high resolution density studies an atomic model of the studied object usually exists and might be used to calculated values of the lost structure factors. Nevertheless, such structure factors are highly biased towards the model used and do not usually show new features of the real electron density in a crystal, which can differ from the atomic model density. These circumstances require the developing of ‘model-free’ methods of calculation of structure factors not-determined in an experiment. Density modification methods (for a review, see e.g. Podjarny, Rees & Urzhumtsev, 1996, e.g.) provide ways to do this.

 

 

3. ‘Soft’ solvent flattening

 

            The outlines of the procedure used are general for iterative density modification methods. Each cycle of the procedure consists of the following steps:

• the synthesis is calculated with the current set of structure factors (the weighted MAD-phased synthesis is used at the first step of the procedure);
• the modification of the synthesis is performed;
• new values of structure factors are calculated from the modified synthesis;
• a new set of values of structure factors is obtained combining calculated phases with the observed magnitudes (if these latter are known), and taking both the calculated magnitude and phase for reflections with unknown magnitudes.

The last step is different from the usual one for unmeasured reflections, for which the whole calculated structure factor is used to update the current values.

In our tests we applied the density modification based on new type of density flattening in the solvent region. The solvent density flattening belongs to the oldest methods of density modification (Bricogne, 1974; Wang, 1985) and is one of the most frequently used tools for phase improvement. Nevertheless, the usual goal of its application is phase refinement and only rarely magnitude restoring. The other feature of the usual approach is that the all density in the assigned solvent region is flattened. At the same time there may exist real density features in this region, which are not interpreted yet. Such density might be removed from the maps as a result of the ‘total’ solvent flattening. The procedure discussed below uses a softer type of density modification in the solvent region. It is based on the observation that the small drops in the maps represent usually noise, while real structural features are represented by more extended regions. The modification discussed consists in reducing of density corresponding to sharp narrow peaks, while the larger ‘blobs’ of a density in the solvent region are left unchanged. Such procedure combines the features of traditional density modification methods with the connectivity based phasing (Lunin, Lunina & Urzhumtsev, 1999, 2000).

            Every step of density modification is defined by:

• a chosen cut-off level  in the Fourier synthesis;
• a mask of the molecular region (i.e., every point in the unit cell is assigned either to the molecular region or to the solvent region);
• a limit size  of drops to be ‘erased’.

First, the set of the points in the unit cell with the synthesis values  is analyzed. The goal of the analysis is to find the number and sizes of connected isolated components in this set. If a component has no common points with the molecular region it is considered as a solvent ‘drop’. If the size of this drop is small enough (smaller than ) the density values for all points in this drop are replaced by . All other points in the unit cell keep their previous values.

            The parameters of the modification are generally updated from step to step.

 

4. Test object.

 

            The tests were performed with a high resolution data set for aldose reductase. The crystals of human aldose reductase belong to space group P2₁ and have unit cell parameters a=49.97, b=67.14, c=48.02 Å, b=92.2^o. There is one molecule per asymmetric unit cell with the molecular weigh about 36 kDa. The crystals diffract to 0.66 Å (Lamour et al., 1999; Howard et al., 2000, Sanishvili et al, paper in preparation) and the structure was refined using SHELX (Howard et al, paper submitted) allowing to collect MAD data to 0.9 Å resolution. This data was phased using SHARP (D'Allantonia et al, paper in preparation). Such high resolution allows starting density distribution studies (Guillot et al., 2000), which were carried before mostly with small molecules. Our tests were done at 0.9Å resolution, starting from MAD-phased data set.           

All the theoretically possible reflections in 0.9Å resolution zone were divided in three sets. Set I consisted of reflections with the measured magnitude and the phase determined by MAD-method. Set II consisted of reflections whose magnitudes were measured, but the phases were not determined. Set III consisted of reflections with unmeasured magnitudes. The last was composed mostly from the very low resolution reflections and reflections of the highest resolution shell (see Table 1).

            To calculate a Fourier synthesis, which represents correctly the electron density, it was necessary:

• to refine the phases in Set I;
• to determine the phases in Set II;
• to restore both magnitudes and phases in Set III.

In the first half of our test for reflections in Set II the goal was changed for restoring both magnitudes and phases. Then, the restored magnitudes for Set II were replaced by the observed ones, and the phases only were refined further. The reason for this was that at the first cycles of structure factors improvement the quality of the newly defined phases is very poor (see Fig.4.) and the use of real magnitudes values deteriorated the process. The restored magnitudes were significantly less than corresponding experimental values and this might be considered as a kind of weighting of such reflections.

 

Table 1. Distribution of reflections in resolution shells.

 

 

 

5. The choice of parameters of the modification.      

 

            The same mask of the molecular region was used during the whole test. It was constructed as the joint region formed by spheres with the radius 0.8Å centred at the atomic centres. The influence of the sphere radius on the progress in structure factors restoring will be discussed elsewhere.

            For every cut-off level it is possible to estimate the mean number of points in the ‘blobs’ corresponding to the recognized water molecules. Approximately half of this value was used to set the parameter, which defines the minimal allowed size for the drops in the solvent region.

            In the total, 1000 steps of iterative structure factors improvement were performed. The procedure was divided into several series of 50-100 steps in average. In every series, the cut-off level  decreased step by step varying in the range from 1.5 – 1.2 ‘sigmas’ in first series to 2.2 – 0.45 in last ones.

 

6. Test results

 

The structure factors calculated from the atomic model cannot be considered as the final goal of the restoring because one of the purposes of high resolution density studies is to find the difference between the real density distribution and that calculated from the model. Nevertheless, these values may serve as a good reference values when restoring structure factors. The increase of the correlation of restored structure factors (of the Set II) with the model structure factors is shown at Fig. 4 for different resolution shells. This figure shows that the quality of restored structure factors significantly grows in the course of modification.

 

 

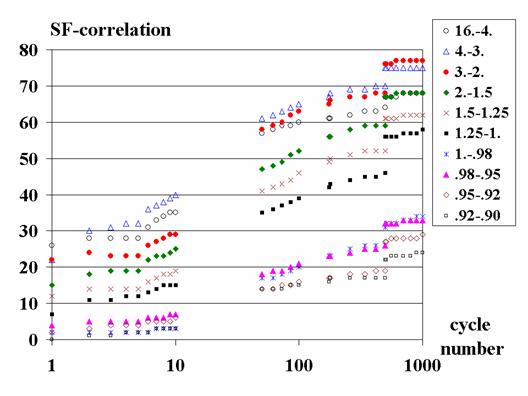
Fig. 4. The growth of accuracy of the restored structure factors for the Set II in the course of the restoring is shown for different shells in the reciprocal space. The shown SF-correlation was calculated as

 

where  are the structure factors calculated from the atomic model and  are calculated from the modified density maps.

 

Sections of Fourier syntheses maps before and after modification are shown in Figs. 5 and 6. In contrast to high cut-off values (Fig.5), the low cut-off maps are sensible to the completeness of the set of structure factors and to the phases accuracy (Fig.6).

 

           

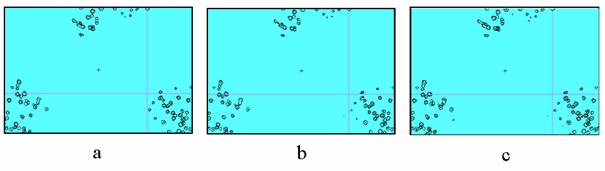
Fig. 5. Aldose reductase Fourier syntheses of 0.9 Å resolution, ‘3-sigma’-cutoff. a) MAD-phased synthesis, 10% of reflections are absent; b) refined phases, 10% of reflections are still absent; c) refined phases, the restored values of structure factors are added for 10% of reflections absent in (a) and (b). The difference between the syntheses is extremely small (see Fig.6 for comparison).

 

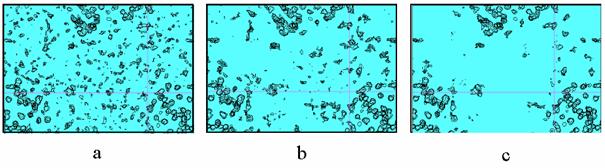
Fig. 6. Aldose reductase Fourier syntheses of 0.9 Å resolution, ‘1-sigma’-cutoff. a) MAD-phased synthesis, 10% of reflections are absent; b) refined phases, 10% of reflections are still absent; c) refined phases, the restored values of structure factors are added for 10% of reflections absent in (a) and (b).

 

 

Fig. 7 shows the decreasing of the number of drops in the solvent region from the MAD-phased synthesis to the final one. 

 

 

 

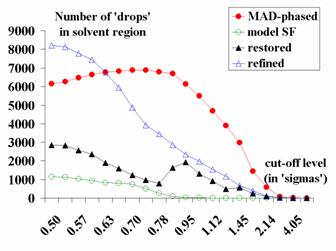
Fig. 7. The number of isolated components in the solvent region for different Fourier syntheses of 0.9 Å resolution: the start MAD-phased synthesis; the synthesis calculated with structure factors obtained from the atomic model (model SF); the synthesis with refined phases, but 10% of reflections absent (refined); the synthesis with all reflection restored and refined (restored).

 

            One of the possible measures of progress in the structure factors restoring is the growth of mean value of restored structure factor magnitudes in comparison with the mean value of the measured magnitudes in the corresponding resolution shell (Fig. 8).

 

 

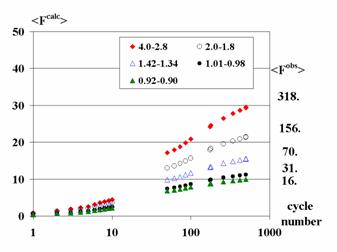
Fig. 8. The growth of mean values of calculated from the modified syntheses magnitudes in the course of the restoring for the Set II (several resolution shells only are shown. The mean values of the observed magnitudes in the considered shells are shown at the right.

 

            The correlation of restored magnitudes with the measured ones (if they are known) may serve as control value too (will be discussed elsewhere).

 

7. Conclusions

 

The test results show that a density modification allows restoring a large number of unmeasured or unphased structure factors. These structure factors improve the image of density distribution map and may be very important in high resolution density studies. Obviously, more advanced density modification techniques may be used as well to improve the power of the method.

 

8. Acknowledgments

 

            This work was supported by CNRS-RAS collaborative program and RFBR grant 00-04-048175. The authors thank Alexandre Urzhumtsev and Claude Lecomte for valuable discussions and possibility to use LCM3B resources. We thank also Andrzej Joachimiak, Ruslan Sanishvili, for their help in collecting the Aldose Reductase data, and Thomas Schneider, Fabio D’Allantonia and George Sheldrick for their collaboration in refinement and MAD phasing.

 

References

 

Bricogne, G. (1974). Geometric Sources of Redundancy in Intensity Data and Their Use for Phase Determination Acta Cryst, A30, 395-405.

 

Guillot, B., Jelsch, C., Muzet, N., Lecomte, C., Howard, E., Chevrier, B., Mitschler, A., Podjarny, A., Cousson, A., Sanishvili, R. & Joachimiak, A. (2000). Multipolar refinement of aldose reductase at subatomic resolution. Acta Cryst. A56 (Supplement), s199.

 

Howard, E. I., Cachau, R., Mitschler, A., Barth, P., Chevrier, B., Lamour, V., Joachimiak, A., Sanishvili, R., Van Zandt, M., Moras, D. & Podjarny, A. (2000). Crystallization of Aldose Reductase leading to Single Wavelength (0.66  Å) and MAD (0.9  Å) subatomic resolution studies. Acta Cryst. A56 (Supplement), s57.

 

Karle, J. & Hauptman, H. (1964). Positivity, Point Atoms, and Pattersons. Acta Cryst. 17, 392-396.

 

Lamour, V., Barth, P., Rogniaux, H., Poterszman, A., Howard, E., Mitschler, A., Van Dorsselaer, A., Podjarny, A. & Moras, D. (1999).Production of crystals of human aldose reductase with very high resolution diffraction. Acta Cryst. D55, 721-723.

 

Langs, D.A. (1998). Reinvestigation of the Use of Patterson Maps to Extrapolate Data to Higher Resolution. Acta Cryst. A54, 44-48.

 

Lunin, V. Yu. (1988). Use of the information on electron density distribution in macromolecules. Acta Cryst. A44, 144-150.

 

Lunin, V. Yu. & Skovoroda, T. P. (1991). Frequency-restrained structure-factor refinement. I. Histogram simulation. Acta Cryst. A47,45-52.

 

Lunin, V. Y., Lunina, N. L. & Urzhumtsev, A. G. (1999). Seminvariant density decomposition and connectivity analysis and their application to very low resolution macromolecular phasing. Acta Cryst. A55, 916-925.

 

Lunin, V. Y., Lunina, N. L. & Urzhumtsev, A. G. (2000). Connectivity properties of high-density regions and ab initio phasing at low resolution. Acta Cryst. A56, 375-382. 

 

Podjarny, A.D., Schevitz, R.W. & Sigler, P.B. (1981). Phasing Low-Resolution Macromolecular Structure Factors by Matrical Direct Methods.,  Acta Cryst. A37, 662-668.

 

Podjarny, A.D., Rees, B. & Urzhumtsev, A.G. (1996). Density Modification in X-Ray Crystallography. In “Methods in Molecular Biology”, 56, 205-226.

 

Urzhumtsev, A. G. (1991). Low-resolution phases: influence on SIR syntheses and retrieval with double-step filtration. Acta Cryst. A47, 794-801.

 

Wang, B.C. Resolution of phase ambiguity (1985). Methods Enzymol., 115, 90-112.

 

Xu, H. & Hauptman, H. A. (2000). On the extrapolation of the magnitudes |E| of the normalized structure factors E. Acta Cryst. A56, 284-287.