Direct Phase Determination by Multi-Beam
Diffraction
Edgar Weckert, Kerstin Holzer, Klaus Schroer
Institut für Kristallographie. Universität Karlsruhe (TH)
Kaiserstr. 12, D-76128 Karlsruhe, Germany
1 Introduction
The knowledge of the three-dimensional structure of a molecule is for many questions in science extremely important since nearly all properties do not depend only on the chemical composition but also on the arrangement of the atoms. For the determination of the three-dimensional structure of such molecules X-ray crystallography plays an important role. However, other methods like NMR (Wüthrich, 1995) gained more importance recently.
The electron density 
(r) of a crystal is periodic in three dimensions. Therefore, the Fourier transform of (r) of the whole crystal
is discrete if the crystal is assumed to be infinite. Owing to this fact it is sufficient to restrict all considerations to one unit cell. The coefficients of the Fourier transform of (r) of the unit cell
are called structure factors and are given by:
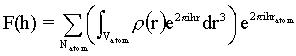(1)
Hereby, h denotes a reciprocal space vector and r are vectors in direct space. The integral in brackets represents the Fourier transform of the electron density of an atom which is commonly called atomic scattering factor f
j(h). F(h) is a complex number which can be separated in modulus and phase
The phase 
(h) of F(h) depends on the origin of the unit cell that was chosen. For an origin shift of 
s the phase (h) will change by 
. The latter term is only valid if absorption is small. This means, an X-ray experiment with
a single reflection delivers only the modulus but not the phase of the structure factors. Therefore, no simple Fourier back transformation
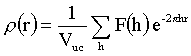(2)
to reveal the electron density of the unit cell is possible. This is well-known as the phase problem of X-ray crystallography. Since the discovery of X-rays many methods have been worked out to surmount the phase problem for both small
and macromolecular structures. For small molecules in general more structure amplitudes 
are available than unknown parameters that are necessary to describe the basic structure, taking also into account that it c
onsists of atoms with a positive electron density everywhere. This is true if reflections up to atomic resolution can be measured. For that case, powerful computer programs are available to solve the structures directly from the measured structure amplitu
des by statistical methods called 'Direct Methods' (Debaerdemaeker, Tate & Woolfson 1988; Sheldrick, 1990; Altomare et al., 1994; Miller, Gallo, Khalak & Weeks, 1994). For structures where no information up to atomic resolution is available additi
onal information has to be provided for a successful solution of the structure. This can be the position of one or more heavy atoms with or without anomalous dispersion contributions (SIR, MIR and MAD) or a significant part of the molecule (molecular repl
acement). All relevant methods have been reviewed in a recent text book (Woolfson and Fan, 1995) and are the object of continuous improvement.
It is the purpose of this contribution to show that under certain circumstances also phase information besides the amplitudes can be obtained directly in a X-ray diffraction experiment. As explained before the phase of a single stru
cture factor has no physical meaning since it depends on the choice of the origin. However, the phase of the product of structure factors whose corresponding reciprocal lattice vectors join to a closed polygon is independent of the origin. Such a quantity
is called invariant. The simplest invariant (besides 
) is a three-structure factor invariant like
(3)
with triplet phase
(4)
Triplet phases play a key role in 'Direct Methods' and it will be shown that they are physically measurable quantities. They consist of a sum of three structure-factor phases. In some cases. however, it is even possible to measure the p hase of a single reflection if one reflection is a seminvariant reflection and if the other two are correlated by symmetry. Seminvariant reflections do not change their phase if one of the symmetrically equivalent origins of the unit cell is chosen. Let < B>(R,t) be the rotational and translational part of a space-group symmetry operation. If triplets of the kind
(5)
can be found the phase of the g reflection cancels since 
. If 
is known, the calculation of 
is stra
ightforward. In 'Direct Methods' triplets like (5) are called 
relationships.
The direct measurement of phase relationships between X-ray reflections is only possible by means of an interference experiment. Hereby, it is necessary to superpose two waves with exactly the same wave vector K. If two waves
with amplitudes A.B and phases 
interfere the resulting intensity is given by
(6)
Equation (6) shows that the intensity depends on the phase difference of the two waves. The idea how this can be achieved in a diffraction experiment by means of a three-beam case was born already in 1949 by Lipscomb.
1
It has been assumed that they have the same K vectors, so the Kr term in the complex exponential functions has already been omitted.
2 Three-beam interference
In a three-beam case there are besides the origin two other reciprocal lattice nodes on the Ewald sphere. This can for example be achieved by the so-called
y-scan technique. Hereby, one reciprocal lattice vector h is brought to its diffraction position. This reflection is considered to be the primary one. By means of a rotation around h a secondary reciprocal lattice node G is turned on to the Ewald sphere. This situation is depicted in Fig. 1. The secondary wavefield with K(g) can in part be diffracted by the reciprocal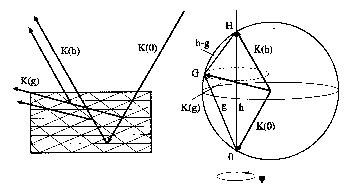
Figure 1: Three-beam case: schematical representation in crystal and reciprocal space with primary reflection h and secondary reflection g; for simplicity all three K vectors are drawn co-planar.< /P>
lattice vector h-g into K(h) direction
2. Therefore, two wavefields are propagated in K(h) direction, the primary one scattered from the h net planes which has a phase shift
(h) and the so-called Umweg wave (detour wave) scattered from g and h-g with the corresponding phase shift (g) + (h–g). According to (6) the intensity in direction K(h) should depend on 
((g) + (h–g)–(h)) and on the amplitudes of the primary and the Umweg wave. This qualitative interpretation already proposed by Lipscomb (1949) is however not sufficient t
o describe the intensities during a three-beam case. For an exact description the dynamical theory of diffraction has to be applied (Colella, 1974; Pinsker 1978; Hümmer & Billy, 1982; Chang, 1984; Chang, 1987: Weckert & Hümmer 1990; Weck
ert & Hümmer, 1997). It is beyond the scope of this article to give a full treatment of this theory for the three- or multi-beam case. For further reading please consult the cited literature. Here only the basic results for understanding the prin
ciples of three-beam diffraction will be summarized. In a perturbational approach (Bethe approximation (Bethe, 1928)) the amplitude in K(h) direction can be written as (Weckert & Hümmer, 1997)
(7)
hereby 
, D denote the amplitudes of the wave fields,
2
The same holds for the wavefield with K(h) via g-h into K(g) direction.
between the crystals electrons and X-rays, r
e = 2.81 10-15 m is the classical electron radius and the resonance terms R(hm) are given by
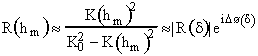(8)
The angle 
represents either 
for h
for hm = g, Ko is the wave vector of the incident radiation inside the crystal. Since K<
/B>(hm) = Ko + hm holds from (8) it is obvi
ous that if absorption is taken into account the resonance terms R(hm) behave like Lorentzians with a phase shift from O to 
if a re
ciprocal lattice vector moves from the inside (|K(hm)| < |Ko|) to the outside (|K(hm)| > |Ko|) of the Ewald sphere. For the further discussion we assume that N 
1 holds in (7). Comparing (7) with (6) the total phase difference for the interference of the two waves can be deduced:
(9)
In Fig. 2 a schematical drawing of amplitude and phase of the resonance term are shown. Suppose the triplet phase of a three-beam case O/h/g is zero: 
= 0°. Then, at the
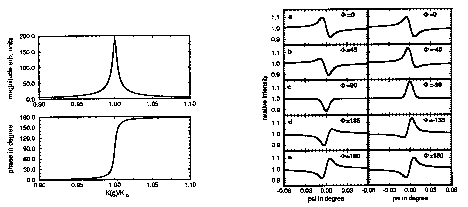
Figure 2: Schematical drawing of amplitude Figure 3: Interference-profiles for different (magnitude) and phase of the resonance term triplet phases R(g) close to the three-beam position.
beginning of the -scan 
= 0 and 
is zero as well. The amplitude of the Umweg wave is small and the two-beam i
ntensity for h is observed. Scanning towards the three-beam position the amplitude of the Umweg wave increases. The primary wave and the Umweg wave interfere in a constructive way which leads to an increase in the resultant amplitude of D(h). Near to the three-beam position shifts rapidly from 0 to 180°, then 
= 180°. That means that the interference becomes destructive and the two-beam intensity is d
ecreased. At the end of the -scan when the amplitude of the Umweg wave decreases, the two-beam intensity is observed again. A calculated profile of this type is shown in Fig. 3a. It reflects the fact that cos[varies from 0 to 180°. The profile forms for other triplet phases 
also shown in Fig. 3 can be explained an
alogously. In Fig. 3 the ratios of the structure factor rnoduli have been chosen appropriately that for example the destructive interference for = 90° is comparable to the constructive interference for = - 90°. However' in general this is not the case since additional symmetric effects which do not depend on the sign of the triplet phase occur which superpose the pure interference effects shown in Fig. 3 (Weckert &
Hümmer, 1990; Weckert, Schwegle & Hümmer, 1993). By comparison of the profiles for two centrosymmetrically related three-beam cases h, g, h-g and 
shown in the left and right column of
Fig. 3, respectively, these Umweganregung (increase) or Aufhellung (decrease) effects can be recognized and eliminated.
3 Experimental
The measured triplet-phase sensitive signal is the change of the intensity of a reflection due to the interference with a second additionally excited one. This means that the rotation around the primary rec
iprocal lattice vector h has to be very accurate as otherwise spurious intensity modulations will occur and spoil any interference pattern. For this purpose a special -circle diffractometer has been constr
ucted which is able to perform a -scan by the rotation of a single axis only. The angular resolution of this diffractometer for those circles that move the crystal is 0.0002 - 0.00005°. In Fig. 4 a schematical dr
awing of the diffractometer is shown. As the detector is mounted on two perpendicular
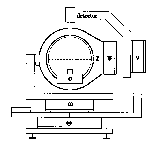
Figure 4: -circle diffractometer
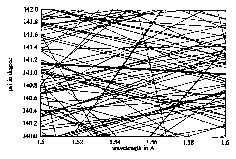
Figure 5: Three-beam positions in dependence on and 
for tetragonal lysozyme with V
circles it can be moved to any direction in the upper half sphere. Thus, also the diffracted intensity of the secondary reflection g during the -scan can be measured which is very import
ant for large structures to obtain the accurate three-beam position.
In Fig. 1 only one secondary reciprocal lattice vector is shown. In reality the number of secondary vectors can be very large. For the crystal structure of a small amino acid at 
= 1.5405 &
Aring; for a full turn in about 6000 three-beam cases occur. This means on average one three-beam case for a 
of 0.05° which is too narrow so that the interference prof
iles of neighbouring three-beam cases would overlap. However, it is possible to find larger gaps for some three-beam cases since the positions are not equally spaced to measure an undisturbed interference profil
e. The positions of different three-beam cases to one particular primary reflection depend very sensitively on the wavelength. Hence, by searching for a suitable wavelength a three-beam case of interest can be s
eparated from neighbours for small and medium size structures. In case of macromolecular structures even by changing the wavelength overlap of different interference profiles can not be avoided. Owing to the fact that the number of weak reflections in mac
romolecular structures is large the wavelength for a given three-beam case with large structure factor moduli can be selected properly that all neighbouring three-beam cases have significant smaller structure factors. Assuming the interesting three-beam c
ase is h, g, h-g with structure factors F(h), F(g) and F(h-g) then it has been shown experimentally as well as theoretically (Weckert, Schwegle & Hümmer, 1993; Weckert & Hümmer, 1997) that the in
terference effect of neighbouring case h, g', h-g' can be neglected if
(10)
The F' are structure factor moduli corrected for polarization. In these cases it is adequate to search for a suitable wavelength that all three-beam cases with q > 0.25 are sufficiently far away from the interesting one. In Fi g. 5 an example for a particular triplet of tetragonal lysozyme is given. Due to the necessity to change the wavelength this experiments require synchrotron radiation which helps also due to its high brilliance to measure the comparable small interference effects.
The crystals used for experimental-phase determination by three-beam interferences are of normal size (0.05 – 1 mm). Protein crystals have been sealed in capillaries together with some mother liquid. The mosaic spread should be as s mall as possible. However, the crystals do not have to be perfect. As long as a crystal consists out of a few larger mosaic blocks whose reflection profile can be separated by the incident radiation
3 three-beam interference experiments with single mosaic blocks are possible. Difficulties arise if the mosaic distribution is smooth and wide.In order to calculate the influence of possible neighbouring triplets and to search for suitable three-beam case an intensity data set as complete as possible is required. For protein crystals also all reflections at low resolution have to be measured.
4 Three-beam experiments with protein crystals
In the past years three-beam interference experiments with various proteins have been carried out (Hümmer. Schwegle & Weckert, 1991; Chang, King, Huang & Gao, 1991; Weckert, Schwegle & H&uu ml;mmer, 1993: Weckert & Hümmer, 1997). The first interference experiments were observed with crystals from sperm whale myoglobine. Later other proteins were investigated like a Fab - fragment (space group: P2
12121, V
280000Å3<
/FONT>), triclinic and tetragonal hen-egg white lysozyme, proteinase K (space group: P43212, V 500000Å3) and trypsin. All experiments were carried out with synchrotron radiation either at beam line C of HASYLAB in Hamburg or from an ESRF bending magn
et (Swiss-Norwegian beamline Grenoble).
In the very beginning wavelengths around 1.54Å were used. In this wavelength range radiation damage is severe. For this reason higher energies ( 1-1.1Å) were selected for more r
ecent experiments. Three-beam interference effects could be observed up to a unit cell size of 1.2 10
3
Using radiation from an ESRF bending magnet, mosaic blocks which are not more than ≈ 0.0 03° inclined towards each other can already be separated.triplet phases per hour can be measured with a 600 
m crystal of tetragonal lysozyme in the resolution range of 3-6 Å. For a 150m crystal of proteinase K in the same resolution range about three triplet phases per hour are still possible. This number can be increased if a more brilliant source is available. The maximum number of triplet phases that could be
measured from a single protein crystals of tetragonal lysozyme was about 150, before the radiation damage was too strong. If crystals of very small mosaicity are available the intensity changes owing to the interference effects are in the order of 5 to 15
%. An example for three-beam interference profiles of tetragonal lysozyme is given in Fig. 6. In Fig. 7 the influence of the radiation damage on a three-beam interference profile is
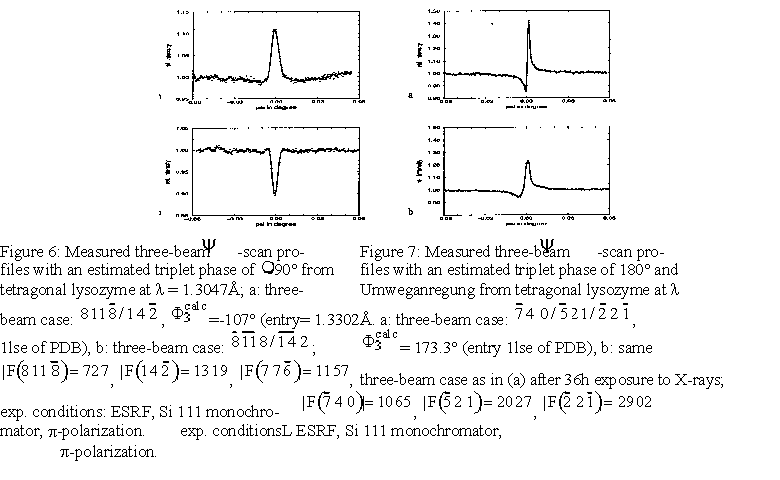
demonstrated. After 36 h of exposure the interference effect is only half as pronounced as for the undamaged crystal.
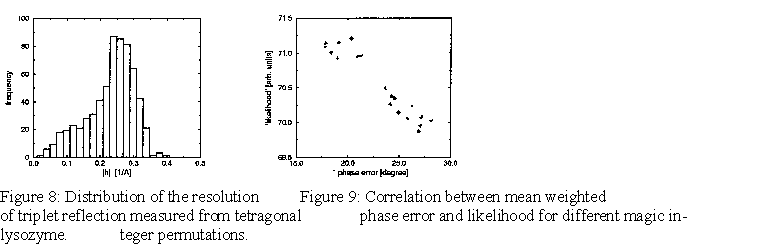
The mean error for the measured triplet phases of all investigated compounds compared to the known structure models was about 20°. In order to test the feasibility of triplet-phase data collection and also to develop a suitable stra tegy it was attempted to measure a larger number of triplet phases from tetragonal lysozyme. Meanwhile. more then 700 triplet-phases have been measured which contain about 630 different single phases. The distribution of the resolution of these phases is shown in Fig. 8. The maximum of this distribution is at about 4Å.
5 Structure determination using experimental phases
In order to apply (2) to calculate an electron-density map single-structure factor phases are needed. which require the choice of an origin. From the 630 reflections two reflections which occur most frequen
tly in different triplets were selected to fix the origin. The phases of this two reflections were taken from the known structure model for comparison. Among the 710 triplet phases were 24 
There should be a number of other possibilities to take advantage of experimental phase information. One of them is certainly in 'Direct Methods' where estimated triplet phases can be substituted by measured ones.
6 Conclusion
It has been shown that the direct determination of triplet phases even from crystals of small proteins is possible provided a stable tune able synchrotron-radiation source is available. The accuracy for the phases that can be achieved seems to be sufficient. Introducing the experimental measured triplets into a maximum entropy based approach is capable to provide single phase which can be used to calculate a map. Compared to other experimental phasing metho ds like MAD the three-beam interference method is slower and crystals of very low mosaic spread have to be used. On the other hand the phase information provided by the three-beam interference method can be obtained from a native protein crystal without i ntroducing heavy atoms. Protein crystals very often show a very small mosaic spread, however, the radiation damage can be quite severe. This seems to be the main problem since the shock freezing method which is successfully applied for intensity data coll ections produces in general a mosaic spread which is too wide for the application of the three-beam interference method.
References
Altomare A., Cascarano, G., Giacovazzo, A., Burla, M., Polidori, G. & Camalli, M. J. Appl. Cryst., 27 (1994) 435
Bethe, H. A. Ann. Phys.(Leipzig), 87 (1928) 55-129
Bricogne, G. & Gilmore, C. J. Acta Cryst., A46 (1990) 284-297
Chang, S. L. Multiple Diffraction of X-rays in Crystals, (1984) Berlin, Heidelberg, New York: Springer Press.
Chang, S. L. Crystallogr. Rev., 1 (1987) 87-189
Chang, S. L.. King, H. E., Huang, M. T. & Gao, Y. Phys. Rev. Lett., 67 (1991) 3113- 3116
Colella, R. Acta Cryst. A30 (1974) 413-423
Debaerdemaeker T., Tate, C. & Woolfson, M. M. Acta Cryst., A44 (1988) 353-357
Hümmer, K. & Billy, H. Acta Cryst., A47 (1982) 841-848
Hümmer, K., Schwegle, W. & Weckert, E. Acta Cryst., A47 (1991) 60-62
Lipscomb, W. X. Acta Cryst., 2 (1949) 193-194
Main, P. Acta Cryst., A33 (1977) 750-757
Miller, R., Gallo, S. M., Khalak, H. G. & Weeks, C. M. J. Appl. Cryst., 27 (1994) 613-621
Pinsker, Z. G. Dynamical Scattering of X-rays in Crystals. (1978) Berlin: Springer Press.
Sheldrick. G. M. Acta Cryst., A46 (1990) 467-173
Weckert, E. & Hümmer, K. Acta Cryst., A46 (1990) 387-393
Weckert, E. & Hümmer, K. (1997) Acta Cryst., A53 (1997) in press
Weckert, E., Schwegle, W. & Hümmer, K. Proc. R. Soc. Lond. A, 442 (1993) 33-46
Woolfson, M. M. & Fan, H.-F. Physical and Non-Physical Methods of Solving Crystal Structures. (1995) Cambridge: Cambridge University Press
Wüthrich, K. Acta Cryst., D51 (1995) 24- 270